How To...
Calculate Voltage Drop In A DC Circuit
Voltage drop is the reduction of voltage as it passes through a conductor to power a device aka load. The factors that affect voltage drop are the resistance of the conductors, and the amount of current moving through the conductors. Ohms Law provides the baseline formula, $V= I x R$.
The Voltage Drop is = to the resistance of the circuit x the current in the circuit.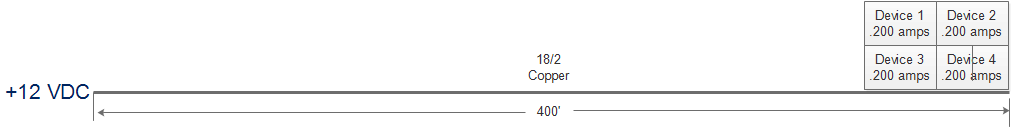
The image above shows a 400' wire run with 4 devices at the end. Each device draws 200mA or .2 Amps for a total of 800mA. Using a wire resistance chart, we know that the resistance for 1000' of 18 AWG wire is 6.385 Ohms.
The formula below requires the total current (.800 Amp) and the length of the wire run (400')
Vdrop (V)= Iwire (A) × (2 × L(ft) × Rwire(Ω/kft) / 1000(ft/kft))
Start with 2 x L (400) = 800 multiply that by x R(6.385) = 5108, then divide that by 1000. You get 5.108 Ohms for your total resistance. Multiple that by I (.800 A) for a total voltage drop of 4.09.
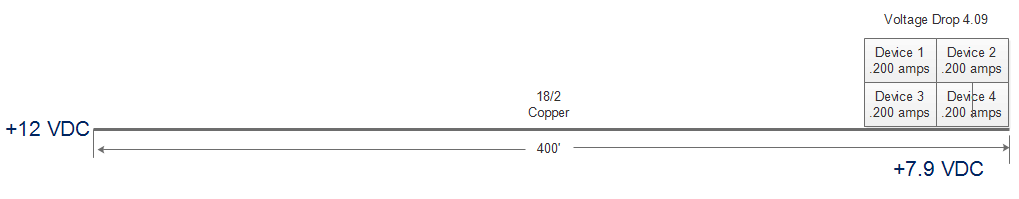
Subtract that from your starting power of 12 Volts and you end with 7.9 VDC. If your devices cannot operate properly at that voltage, you may need to increase the wire gauge to lower the resistance, shorten your wire run, or increase your power at the source.
Distributed Load Circuit
The example above has the entire load at the end of the 400' wire run. If your devices are distributed along the path, the voltage changes as you move down the wire path.

Using the same formula we now only need to factor in the 1st 100' section because we are interested in the voltage at device 1. You still have a .800 A load because that current is present at Device 1.
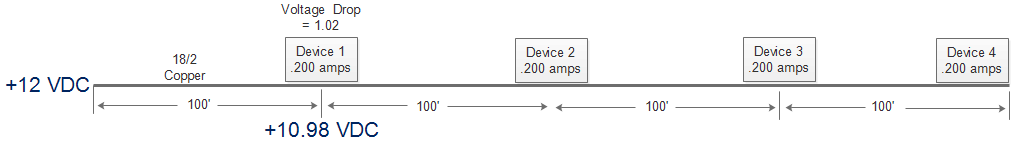
Start with 2 x L (100) = 200 multiply that by x R(6.385) = 1277, then divide that by 1000. You get 1.277 Ohms for your total resistance. Multiple that by I (.800 A) for a total voltage drop of 1.02. 12 - 1.02 leaves a voltage of 10.98 VDC at device 1.
Now we start all over, ignoring device 1 because that current is no longer on the circuit. Instead of 12 VDC, we start with 10.98.
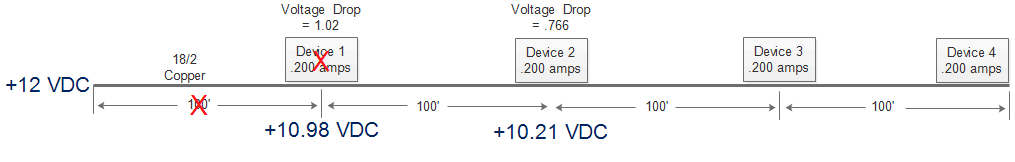
Start with 2 x L (100) = 200 multiply that by x R(6.385) = 1277, then divide that by 1000. You get 1.277 Ohms for your total resistance. Multiple that by I (.600 A) for a total voltage drop of 1.02. 12 - 1.02 leaves a voltage of 10.98 VDC at device 1. Notice the only value that changed was the current which dropped to .600 A.
Now we start all over, ignoring devices 1 and 2 because that current is no longer on the circuit. Instead of 10.98 VDC, we start with 10.21.
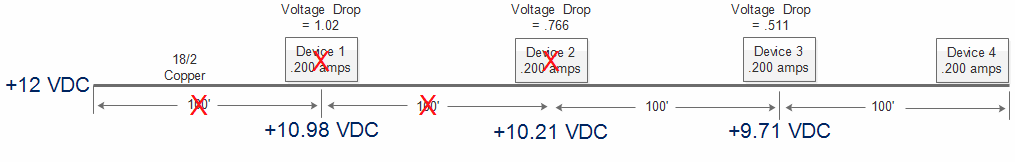
Start with 2 x L (100) = 200 multiply that by x R(6.385) = 1277, then divide that by 1000. You get 1.277 Ohms for your total resistance. Multiple that by I (.400 A) for a total voltage drop of .511. 10.21 - .511 leaves a voltage of 9.71 VDC at device 3. Notice the current which dropped to .400 A.
Now we start all over, ignoring devices 1, 2. and 3 because that current is no longer on the circuit. Instead of 10.21 VDC, we start with 9.71.
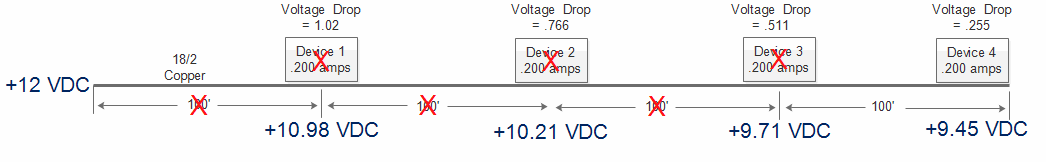
Start with 2 x L (100) = 200 multiply that by x R(6.385) = 1277, then divide that by 1000. You get 1.277 Ohms for your total resistance. Multiple that by I (.200 A) for a total voltage drop of .255. 9.71 - .255 leaves a voltage of 9.45 VDC at device 4. Notice the current which dropped to .200 A.
By using the distributed load method you gain a more realistic idea of what your voltage drop will be. If you calculated the distributed system using the first (all at the end) method and you were OK with the voltage value you calculated, then you know Voltage Drop would not be an issue.